3 Chapter 1 c2 1 2 ( √ 3 ) 2 4 ⇒ c √ 4 2 d2 1 2 2 2 5 ⇒ d √ 5 e2 1 2 (√ 5 ) 2 6 ⇒ e √ 6 f 2 1 2 ( √ 6 ) 2 7 ⇒ f √ 7 g2 1 2 ( √ 7 ) 2 8 ⇒ g √ 8 2 √ 2 The length of "c" is a rational number 13 Since the length of the carpet equals the sum of the heightSolve your math problems using our free math solver with stepbystep solutions Our math solver supports basic math, prealgebra, algebra, trigonometry, calculus and moreValue of Sin 15 degree = (√3 1) / 2√2 Alternative Method to Determine Sin 15 Values The angle 15 0 can be written as the difference of 45 0 and 30 0 Sin 15 0 can be written as Sin (45 0 30 0) The value can be determined by using the difference formula Sin (A B) = Sin A Cos B Cos A Sin B Sin (45 0 30 0) = Sin 45 0 Cos 30 0
If X 3 2 2 Then What Is The Value Of X 1 X Quora
Value of (3^2)^2
Value of (3^2)^2-I = 6 (√2 – 1) 2√3 – 2√2 6 – 3√3 I = 1 – √2 – √3 Was this answer helpful?The value of √(3 2√2) is A √3 √2 B √3 √2 C √5 √6 D none of these asked Apr 16 in Number System by Vaibhav01 ( 395k points) rationalisation




How To Do This Question X 3 1 2 Find Value Of 4x 3 2x 2 8x 7 Mathematics Topperlearning Com 4r5prjll
Harsha Vardhan · BTech in Electronics and Communication Engineering & Internet of Things (IoT), National Institute of Technology, Warangal ( Expected 22) Sin75 = sin (30 45) Sin75 = sin30 * cos45 cos30 * sin45 Sin75 = 1/2 * 1/√2 √3/2 * 1/√2 Sin75 = (√3 1)/2√2 52 views answered by ajoy yadav (248k points) selected by vikash gupta Best answer (i) (3 √3) (2 √2) We need to apply distributive law to find value of (3 √3) (2 √2) (3 √3) (2 √2) = 3 (2 √2) (3 (2 √2) = 6 3√2 2√3 √6√ 3 2 √ 2, we find x = − √ 2 √ 3, y = √ 2 A negative value for λ is impossible, since otherwise x and y would be negative, Page 3 of 4 A Sontag Math 5 HWK a Solns continued which makes no sense in this problem Using λ = 2 r pq A, then, we find x = q s A pq = s Aq p feet y = p s A pq = s Ap q feet It's apparent from the realworld problem that these
Cosine Sum Identity cos( AB)=cos Acos B−sin Asin B Example 351 Findtheexactvaluesofcos105 andcos π 12 Solution cos105 =cos(60 45 ) =cos60 cos45 −sin60 sin45 1 2 √ 2 2 − √ 3 2 √ 2 2Simplify the following expression ( 3 √ (3)) ( 2 √ (2)) Simplify the following expr Free Online Scientific Notation Calculator Solve advanced problems in Physics, Mathematics and Engineering Math Expression Renderer, Plots, Unit Converter, Equation Solver, Complex Numbers, Calculation History
(3√32√2)(3√32√2) = (3√3)^2 (2√2)^2 = 278 = 21 and we have 4(3√32√2) 3(3√32√2)21 12√38√29√36√221 (21√32√2)/21 = √3 (2/21)√2 Though why you would want to go through any of that is unclear, since you apparently want to use the approximate values of √2 and √3 Just use your calculator to evaluate the original expression The value of √(3 2√2) is A √3 √2 B √3 √2 C √5 √6 D none of these asked Apr 16 in Number System by Vaibhav01 ( 395k points) rationalisationI need help with




If X 3 2root2 Then Find The Value Of X4 1 X4 Brainly In
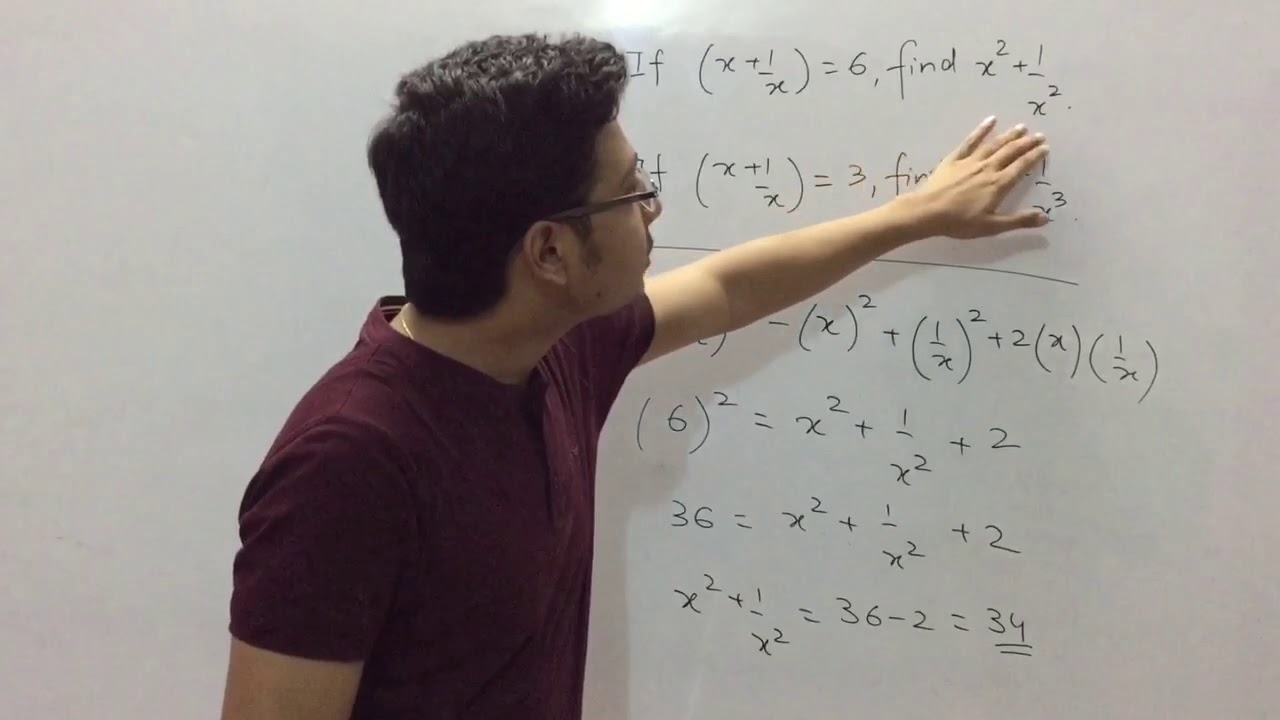



If X 1 X 3 Then Find The Value Of X 3 1 X 3 If X 1 X 6 Find X2 1 X2 Polynomials Class 9 Youtube
√3 2 √2 2 1 2 0 Work out the exact value of x Work out the exact value of x Work out the size of angle y Tangent State the value of tan for 0⁰ 30⁰ 45⁰ 60⁰ 0 √ √3 3 1 3 Work out the exact value of x Work out the exact value of x Work out the size of angle y Challenge Questions Show that cos 30° 4 sin 60° 3 2 tan 60° can be written in the form √𝑘 where 𝑘is anIf 2 = 1414, 3 = 1732, then the value of 3 3 −2 2 4 3 3 2 2 3 is 1063 If true then enter 1 and if false then enter 0 If 2Use a doubleangle or halfangle identity to find the exact value of each expression cos θ = 4/5 and 270° < θ < 360°Find sin 2θ answer choices 1/5 24/2524/2525/24 s Question 2 SURVEY 900 seconds Q Use a doubleangle or halfangle identity to find the exact value of each expression sin θ = −3/5 and 3π/2 < θ < 2π Find tan θ/2 answer choices 1/3√3 √3/31



What Is The Value Of 3 2 2 Quora



If X 3 2 2 Then What Is The Value Of X 1 X Quora
Find the value of cos 15 ° Solution Write the given angle 15 ° in terms of sum or difference of two standard angles Then, 15 ° = 45 ° 30 ° cos15 ° = cos (45 ° 30 °) cos 15 ° = cos 45 ° cos 30 ° sin 45 ° sin 30 ° Using the above trigonometric ratio table, we have cos 15 ° = (√2/2) ⋅ (√3/2) (√2If α, β are zeroes of x 2 − 4 x 1, then the value of α 1 β 1 − α β is View solution If α and β be the roots of the equation x 2 p x q = 0 then the equation whose roots are α 2 α β a n d β 2 α β isThen, n ∑ k=1 k·k!




What Is The Solution Of 2 3 2 3 Quora



What Is The Value Of 3 2 2 3 3 2 2 3 Quora
4 (12) Upvote (17) Choose An Option That Best Describes Your Problem Answer not in Detail Incomplete Answer Answer Incorrect Others Answer not in Detail(D)(n!) 2 −1 (E) None of the above 1 2 Notice that k·k!=√3/2√2–1/2√2 =√3–1/2√2 151 views View upvotes 2 Share Sabir Ali Studied at SSC CGL 18 Use formula Sin(AB) = SinACosBCosASinB Use A=60° & B=45° Sin15° = Sin(60–45)° = Sin60°Cos45° Cos60°Sin45° Sin15° = (√3/2)*(1/√2) (1/2)*(1/√2) = (√3–1)/2√2 Values used are in respective order Sin60°= √3/2, Sin45°=Cos45°= 1/√2



If A 3 2 3 2 And B 3 2 3 2 Then How Do I Find A 2 B 2 Quora




Simplifying Radical Expressions
Answered May 17 Author has 92K answers and 63M answer views Let the value of √ (3–2√2) is (√x √y), accordingly (√x √y)^2 = (3 2√2) or, x y 2√ (xy) = 3 2√2 NCERT Solutions for Class 10 Maths NCERT Solutions for Class 10 Maths Chapter 1 NCERT Solutions for Class 10 Maths Chapter 2 NCERT Solutions for Class 10 Maths Chapter 3 NCERT Solutions for Class 10 Maths Chapter 4 NCERT Solutions for Class 10 Maths Chapter 5 NCERT Solutions for Class 10 Maths Chapter 6Solution(4/3√3–2√2)(3/3√32√2) = 4(3√32√2) 3(3√3–2√2) /(3√32√2)(3√3–2√2) = 12√3 8√2 9√3 6√2 / (27–8) = 21√3 2√2 /19 =21×1732 2×1414 / 19 = 28 / 19 = 390/19 = 63




Square Root Of 2 Wikipedia



Q Tbn And9gcqnfu4xl8biccm2lo1vb Feimstd8kb0bjmfkodcsezzh7idds Usqp Cau
The value of the upper quartile is It is the term in the distribution D7 is the element It is equal to The Peo is the element So, Po is equal to The median data which is also the Q2, Ds, and Pso is Therefore, 50% of students who have internet access per class is less than or equal toPlace Value of a Number This selection will help you to find what the place value is of a particular digit in a number Type your number here, then click "Take my number," and we ' ll go from there Type your number here Quick!√ 3 2 √ 2 Answer is Problem 3 Calculate the expression 1·1!2·2!3·3!n·n!
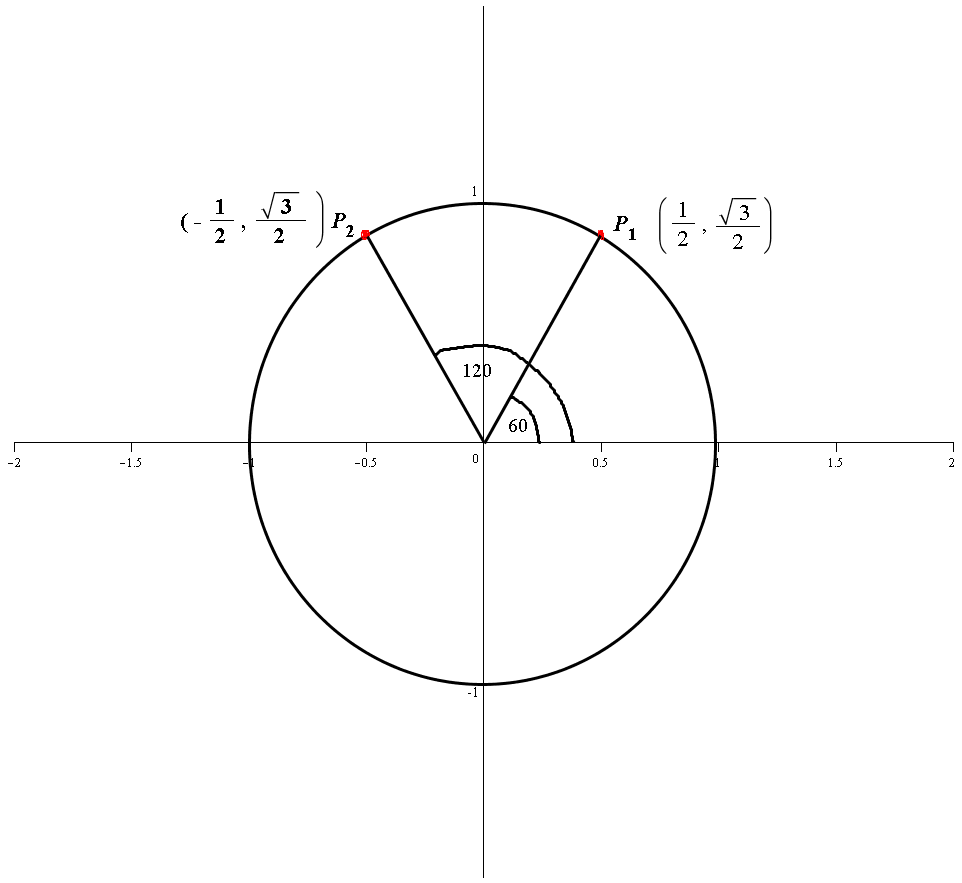



If The Point X Sqrt 3 2 Is On The Unit Circle What Is X Socratic
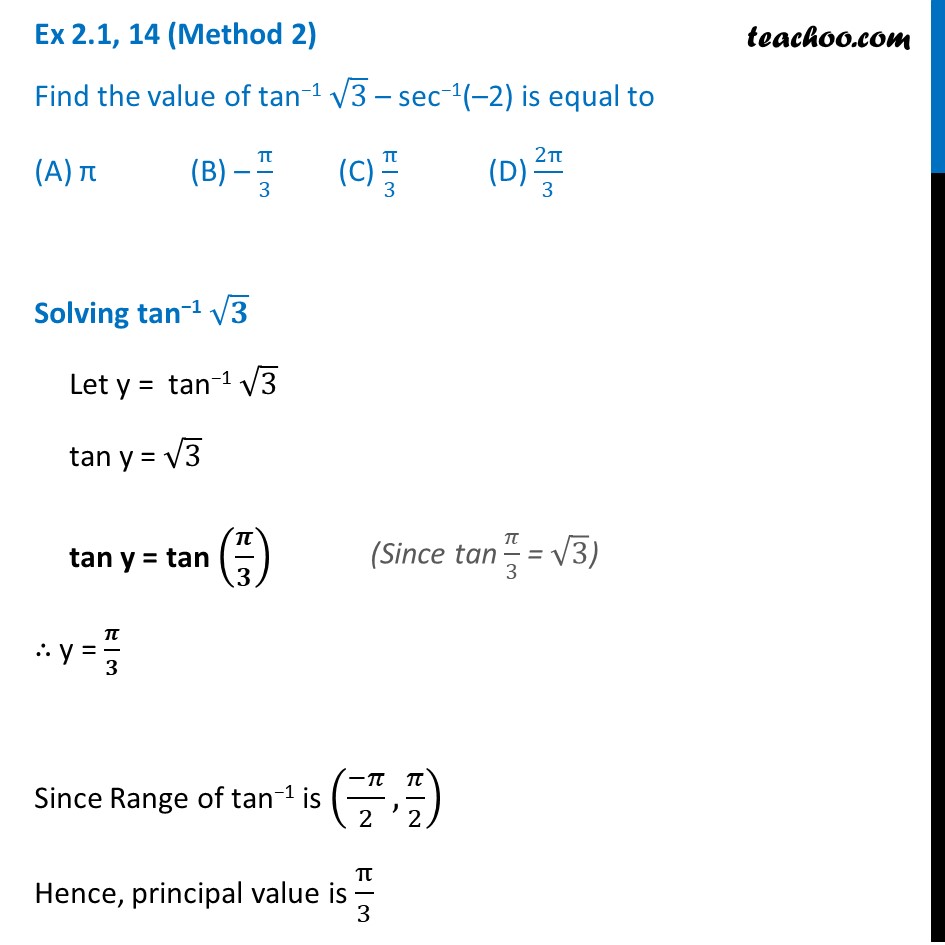



Ex 2 1 14 Find Value Of Tan 1 Root 3 Sec 1 2 Ex 2 1
Substitute the value of sin 30, sin 45, cos 30 and cos 45 degrees, then equation (2) will becomes Cos (45 – 30) = 1/√2 √3/2 1/√2 1/2 Cos 15 = √3/2√2 1/2√2 Cos 15 = √31/2√2 Was this answer helpful? Find an answer to your question Find the value of tan 75 degree an9upa7jeevsounnu an9upa7jeevsounnu Math Secondary School answered Find the value of tan 75 degree 2 See answers ROYALJATT ROYALJATT Tan(75°) = tan(45° 30°) = tan(45°) tan(30°)/1 tan(45°)tan(30°) = 1 1/√(3)/(1 1/√(3) tan(75°) = (√(3) 1)/(√(3) 1) Alanaaron AlanaaronFind the value of cos15 ° Solution First, we have to write the given angle 15 ° in terms of sum or difference of two standard angles So, we have 15 ° = 45 ° 30 ° cos15 ° = cos (45 ° 30 °) cos15 ° = cos45 ° cos30 ° sin45 ° sin30 ° Using the above trigonometric ratio table, we have cos15 ° = (√2/2) x (√3/2) (√2/2




Square Root Of 2 How To Find The Square Root Of 2



What Is The Solution Of 2 3 2 3 Quora
The value of 2 2√2 A is 2 B is greater than 2 C is 1 D cannot be found 1 mark 6 °The value of sin105 is A √3 2√2 B 1−√3 2√2 C √31 2√2 D √3 √2 1 mark 7 The sum of the finite series 123 234 345 ⋯ 𝑛𝑛(𝑛𝑛 1)(𝑛𝑛 2) is A 2𝑛𝑛(𝑛𝑛 1)(2𝑛𝑛 1)⁄6 B 𝑛𝑛(𝑛𝑛 1)(𝑛𝑛 2)(𝑛𝑛 3)⁄4 C When we substitute the value of √5 in equation 2 –√5, we get, 2√5 = 2√5 = Since the number, – , is a nonterminating and nonrecurring, Therefore, 2 –√5 is an irrational number (ii) (3 √23) √23 (3 √23) –√23 = 3√23–√23 = 3 Since, the number 3 is rational number Therefore, (3 √23) √23 is rational (iii) 2√7SolutionShow Solution √2 = 14 and √3 = 17 `1/ (√3 √2 )` = `1/ (√3 √2 ) xx (√3 √2)/ (√3 √2)` = ` ( √3 √2 )/ (√3)^2 (√2)^2` = ` √3 √2 / ( 3 2 )` = √3 √2 = 17 14 = 31



What Is The Value Of A And B If 5 2 3 7 4 3 A B 3 Quora
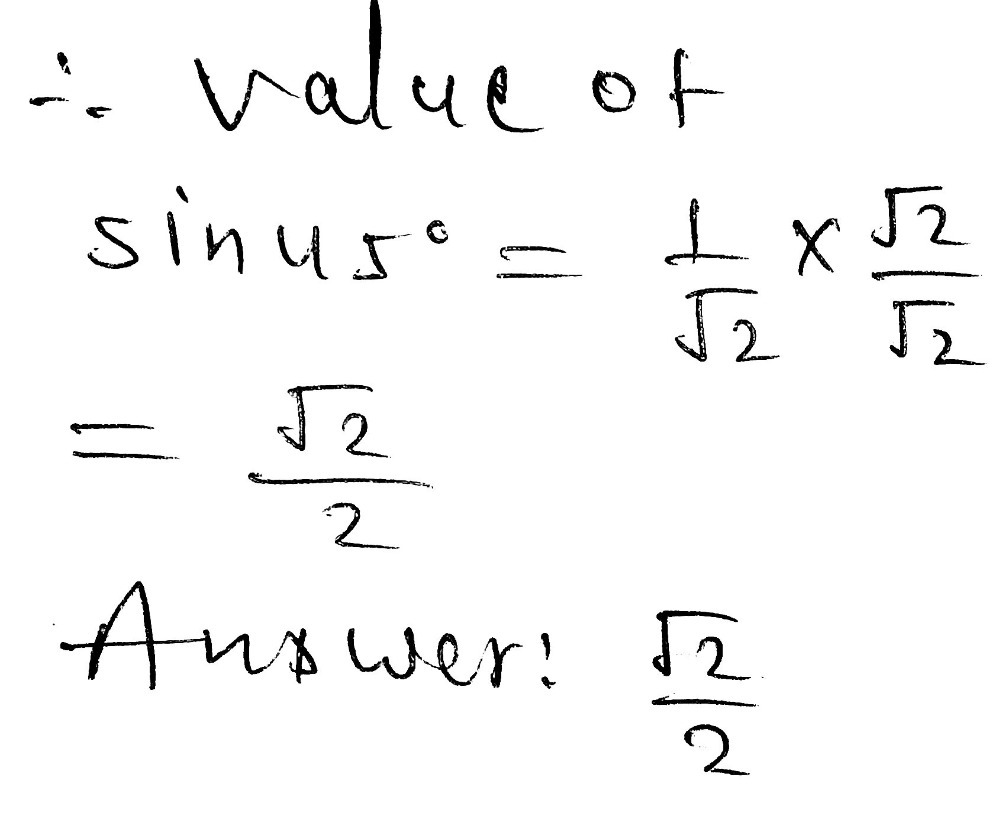



Which Of The Following Is The Value Of Sin 45 1 Gauthmath
The sum and difference formulas are good identities used in finding exact values of sine, cosine, and tangent with angles that are separable into unique trigonometric angles (30°, 45°, 60°, and 90°) Shown below are the sum and difference identities for trigonometric functions Addition Formula for Cosine cos (u v) = cos (u) cos (vSin 45 Degrees The value of Sin 45 degree in decimal form is Sine is considered as one of the most important functions in trigonometry as it is used to find out the unknown values of the angles and length of the sides of a rightangle triangleCBSE Class 9 Maths Number Systems MCQs Set B with answers available in Pdf for free download The MCQ Questions for Class 9 Number System with answers have been prepared as per the latest 21 syllabus, NCERT books and examination pattern suggested in Standard 9 by CBSE, NCERT and KVS Multiple Choice Questions for important part of exams for Grade 9 Number




How To Multiply Square Roots 8 Steps With Pictures Wikihow



1
Davneet Singh is a graduate from Indian Institute of Technology, Kanpur He has been teaching from the past 10 years He provides courses for Maths and Science at Teachoo The value of √(3 2√2) is A √3 √2 B √3 √2 C √5 √6 D none of these asked Apr 16 in Number System by Vaibhav01 ( 395k points) rationalisationSimply give the number in the specified input box and hit the calculate button to find the cot inverse value much easier and faster Cot Inverse Calculator cot1 Calculate Cot Inverse Calculator Are you looking for a smart tool that calculates the cotangent inverse of a real number or fraction within no time?




Simplifying Square Root Expressions Video Khan Academy
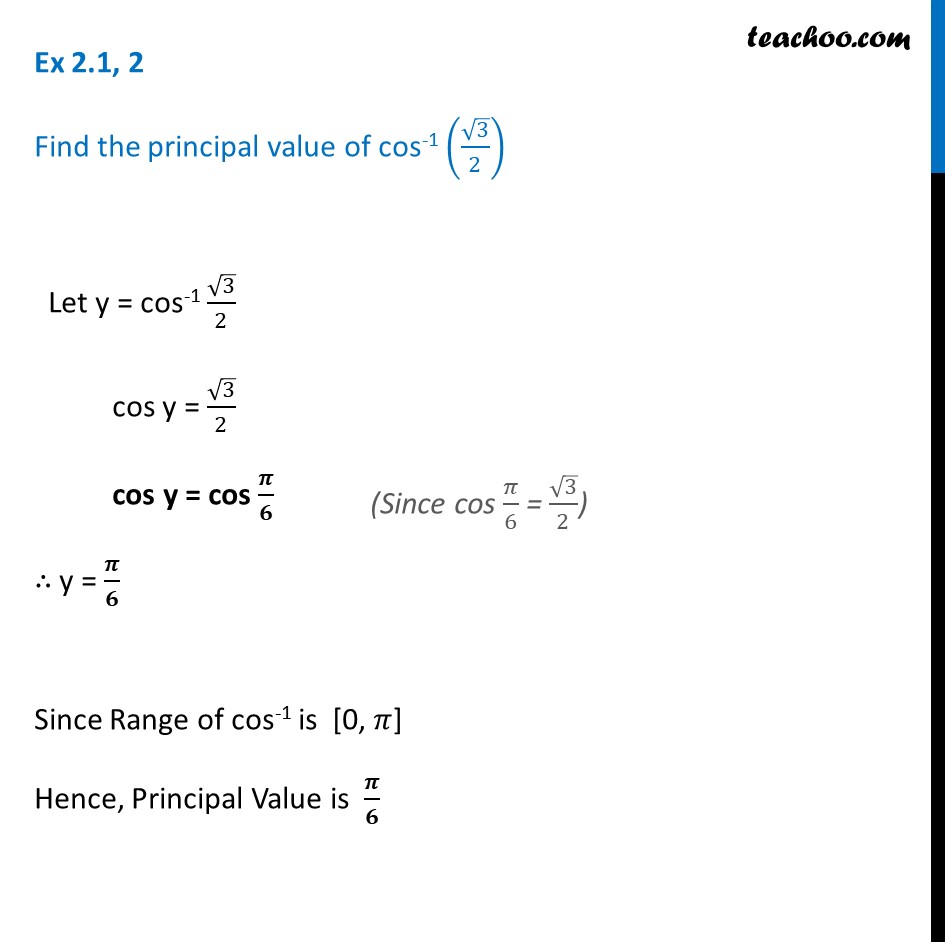



Ex 2 1 2 Find Principal Value Of Cos 1 Root 3 2 Class 12
PR= 1 unit Extend side QR to T such that PR= RT Join PT Calculate the exact measure of angle T Determine the exact value of lengths needed to Algebra 2 Find the exact value of cos 315 degrees answers 3/2, 1/2,2/2, 2/2 Trig(3 all with work) 1)Find the exact value of cos 105 by using a halfangle formula A)sqrt 2 sqrt 3 /2 B)sqrt 2 sqrt 3 /2 C)sqrt 2 sqrt ️ solutionto find the value of this question (√3 2√2) (√3 2√2)= √3 2√2 √3 2√2= √3 √3 2√2 2√2= 0 4√2= 4√2 answer= 4 × √2= 4 × (1414) a Search Its collection of trigonometric values of various standard angles including 0 °, 30 °, 45 °, 60 °, 90 °, sometimes with other angles such as 180 °, 270 ° and 360 ° Trigonometry Table has trigonometric functions – sine, cosine, tangent, coscent, secant, cotangent These ratios can be abbreviated as sin, cos, tan, cosec, sec, and cot The value of trigonometric ratios of standard



What Is The Square Root Of 21 3 8 6 3 6 7 24 56 2 21 Quora




Simplify Each Of The Following Expressions I 3 3 2 2 Ii 3 3 3 3 Iii 5 2 Brainly In
Then, you have arrived the correct place and our calculator is the best tool thatAnswered 1 year ago Author has 1K answers and 211K answer views sec 165°,it is also written as »sec165°=sec (1°45°) »sec165°=sec (90°75°), sec (90°A)=–cosecA »sec165°=–cosec75°,cosec75°=2√2/√3 1 sec165°=–2√2/ (√3 1),Ans I hope it is helpful to you 413 views · The value of √(32√2) is A √2 1 B √2 1 C √3 √2 D √3 √2




If X 2 3 Find The Value Of X 2 1 X 2 If X 2 3 Find The Value Of X2 1 X2 Polynomials Class 9 Youtube




2 3 3 2 2 3 A B 6 Find A And B Brainly In
= n ∑ k=1 ((k1)!−k!)=(n1)!−1 Answer is (B) Problem 4 What is the minimum value of f(n)=2n2 3n1, where n can be any real number?0 (0) Upvote (0) Choose An Option That Best Describes Your Problem Answer not in Detail Incomplete Answer Answer Incorrect Others Answer not in Detail Incomplete Answer Answer Incorrect Others Thank you Your Feedback will Help us Serve you better Related Links The Value Of(A) 1 (B) −3 4




A Cambridge Interview Problem Sqrt 3 2sqrt 2 Youtube




4 What Is The Value Of X A 3 B 3 Square Root O Gauthmath
If√2 = 1 414,√3 =1 732 then find the value of 4 3 3√3 2√2 3√3 2√2 Maths Coordinate GeometryFind the exact values of each expression a os 75 cos 1 Find the exact values of each expression a os75° cosc b oscosc π12 c in105° sins d os15° cosc e an 75° tan t Solutions a Notice that Since we know the exact value of sine and cosine at 5° 5° 0° 7 = 4 3 , we use the Addition Formula for Cosine to get 5° and 30° 4 os



Square Root Of 2 Wikipedia




If X 3 2 2 Find X 4 1x 4




Solve 8 0 2 3 0 6 2 3x For The Value Of X




If X 3 2 2 Find X 3 1 X3 Brainly In




A 3 2 2 Then The Value Of A 1 2 A 1 2 Can Foundation Brainly In



Simplify 3 2 2 Evaluate 53 10 6 Cambridge Interview Maths Questions Sqrt 3 2 Sqrt 2 Youtube



What S The Value Of 2 And 3 Quora




How To Do This Question X 3 1 2 Find Value Of 4x 3 2x 2 8x 7 Mathematics Topperlearning Com 4r5prjll




Multiplying And Dividing Radical Expressions
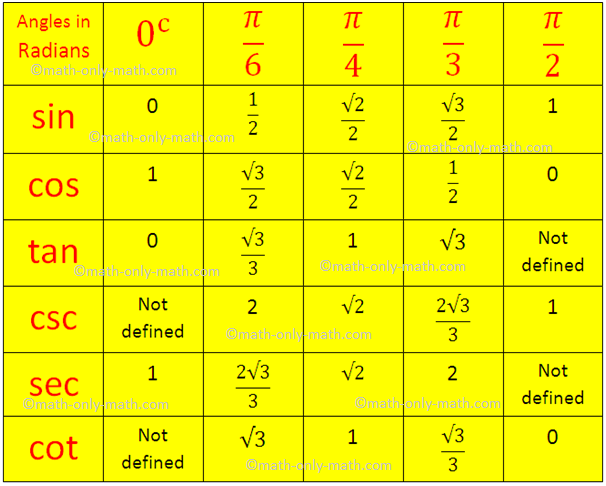



Trigonometrical Ratios Table Trigonometric Standard Angles Standard




What Is The Value Of 4 Root 3 Root 2 Square In Exponential Form Brainly In




Multiplying And Dividing Radical Expressions



Inverse Cos Sqrt 3 2 Youtube




Rd Sharma Class 9 Solutions Maths Chapter 3 Rationalisation




How To Write Exact General Solution Sinx Sqrt 3 2 For Trigonometric Equation Youtube




Algebra Toughest Questions Algebra Teaching Mathematics




If X 3 2 Root 2 Find The Value Of Root X 1 Root X Brainly In




How To Solve Surds 2 Four Essential Surd Techniques Suresolv



What Is The Principal Value Of Cos 1 3 2 Sarthaks Econnect Largest Online Education Community
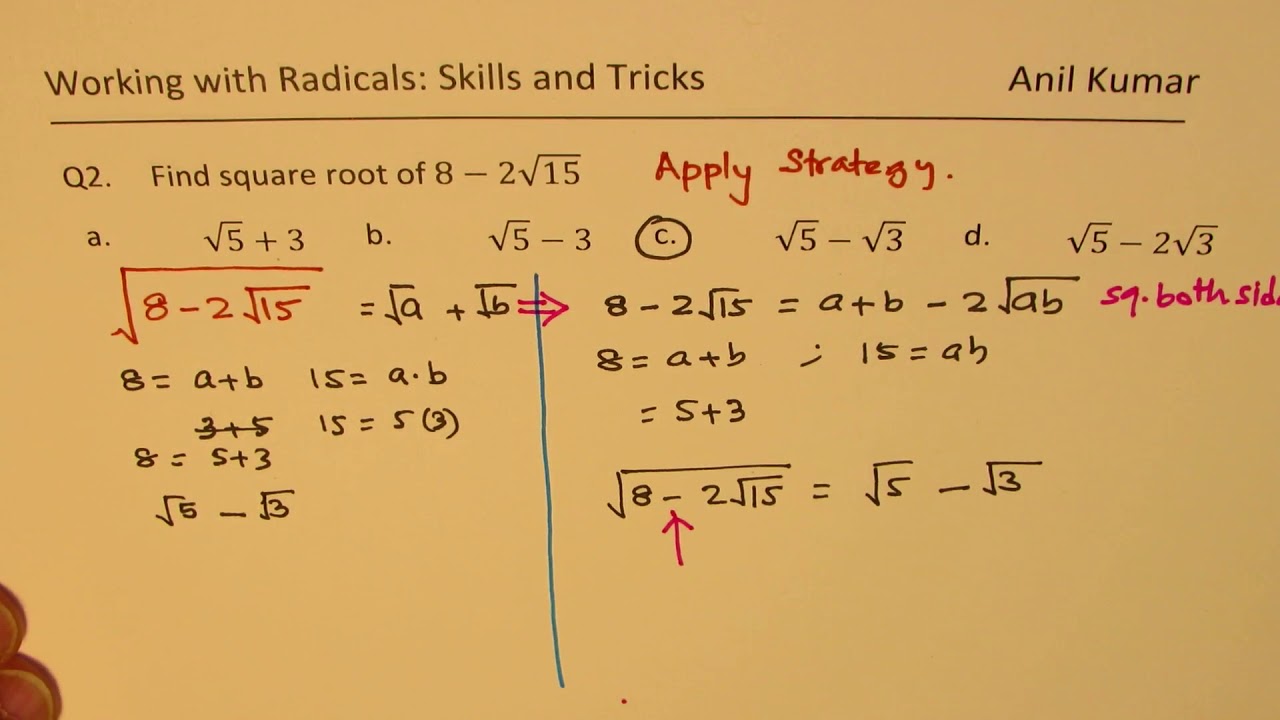



Strategy And Trick To Find Square Root Of 8 2 15 Radical Expressions Youtube



Find The Value Of A And B If 5 2 3 7 4 3 A B 3 Studyrankersonline




Simplifying Square Root Expressions No Variables Video Khan Academy




What Is The Solution Of 2 3 2 3 Quora




Find The Value Of Square Root 2 Root 3 2 Root 3 If Root 3 1 73 Brainly In
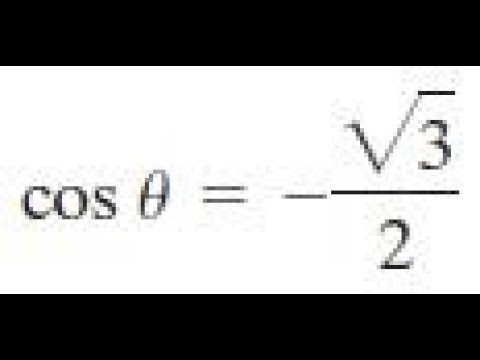



Cos X Sqrt 3 2 Give The General Formula Solutions Youtube




Find The Value Of 6 3 2 2 3 Brainly In




What S The Value Of 2 And 3 Quora




Introduction To Complex Numbers And Complex Solutions



Q Tbn And9gcssp2 7fyz3j2ny005jbktvc79izmavixzm H7ylgukjb6hwogm Usqp Cau




Determine The Value Of 1 Sqrt 1 Sqrt 2 1 Sqrt 2 Sqrt 3
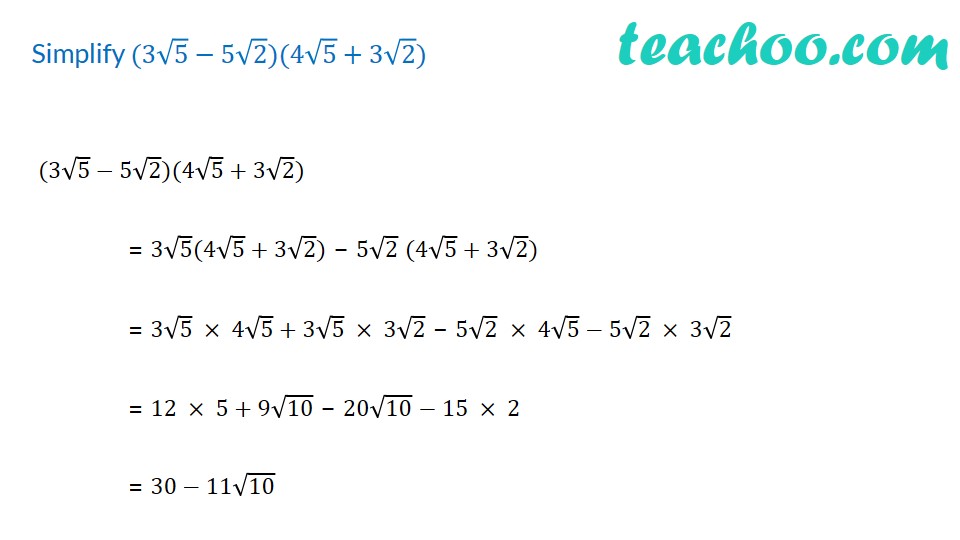



Simplify 3 5 5 2 4 5 3 2 With Video Teachoo Rationalising
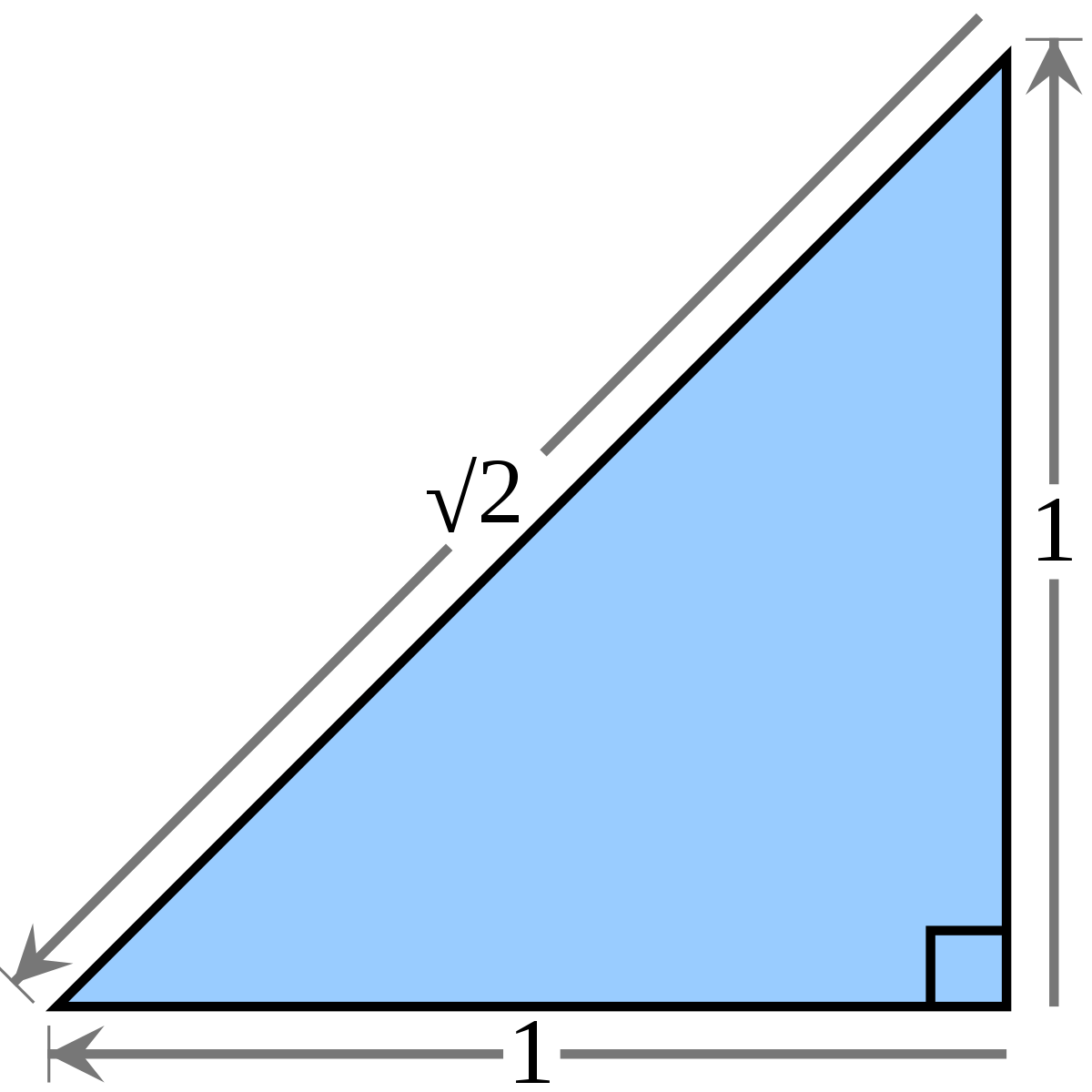



Square Root Of 2 Wikipedia




If X 3 2 Root 2 Find The Value Of X 1 X Youtube



If A 3 2 3 2 And B 3 2 3 2 Then How Do I Find A 2 B 2 Quora



What Is The Value Of 3 Quora



What Is The Value Of 3 2 2 Quora




What S The Value Of 2 And 3 Quora




What Is The Answer To 2 3 Quora




The Value Of Expression X 4 8x 3 18 X 2 8x 2 When X 2 Sqrt 3
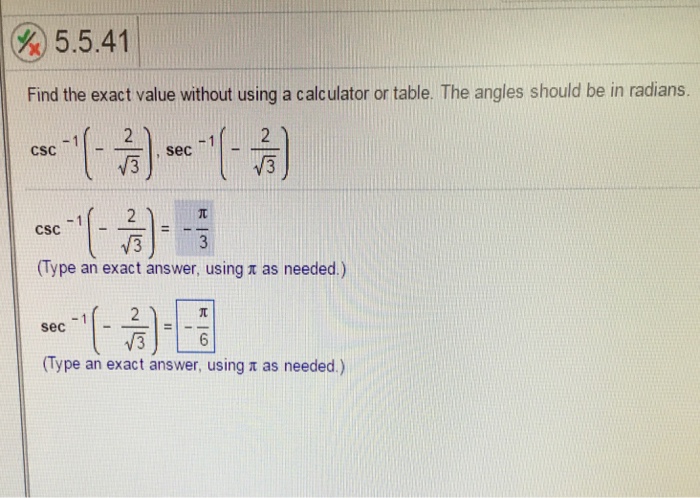



What Is The Answer To Sec 1 2 Square Chegg Com
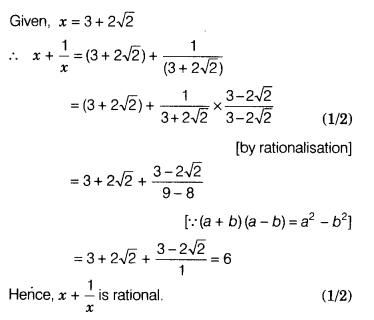



If X 3 2 2 Then Check Whether X 1 X Is Rational Or Irrational Cbse Class 9 Maths Learn Cbse Forum




If X 3 8 Find The Value Of X 2 1x 2




Rationalize 1 7 3 2 Maths Questions




Find Value Of A And B 3 Radic 6 Radic 3 Radic 2 A B Radic 3 Mathematics Topperlearning Com Lujm5xqq




Value Of Root 3 Find The Square Root Of 3



Q Tbn And9gcqplaucvqixlmspmlitmr7v Qihec7cf8btubly6oox8xl7azkj Usqp Cau
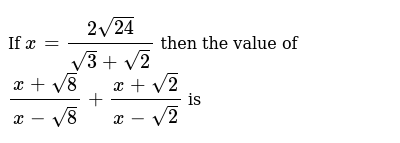



If X 2sqrt 24 Sqrt 3 Sqrt 2 Then The Value Of X Sqrt 8




Square Root Of 2 Wikipedia




If Sqrt 2 1 414 And Sqrt 3 1 732 Find The Value Of Sqrt 72 Sqrt 48 Youtube



If X 3 2 2 Then What Is The Value Of X 1 X Quora
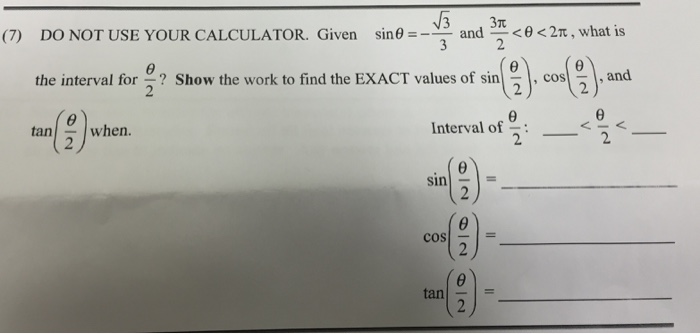



Given Sin Theta Square Root 3 3 And 3 Pi 2 Chegg Com




Solving Quadratics By Taking Square Roots Examples Video Khan Academy
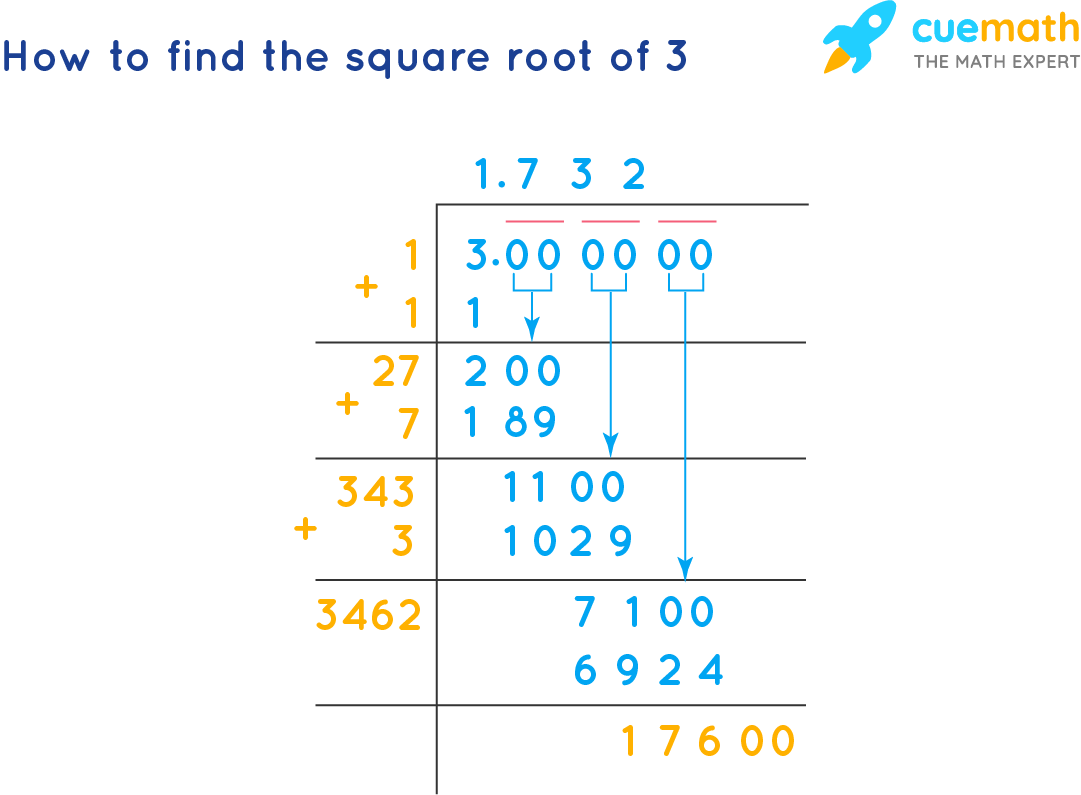



Square Root Of 3 How To Find The Square Root Of 3




Solve 3 5 3 2 5 A 5 B Youtube




If X 3 2 3 2 And Y 3 2 3 2 Then Find The Value Of X Y Please Answer Fast Brainly In




Find The Value Of B In 2 3 3 2 2 3 2 B 6 Brainly In



What Is The Value Of 4x 3 2x 2 8x 7 If X 3 1 2 Quora



What Is The Value Of Math Sqrt 6 Sqrt 6 Sqrt 6 Infty Math Why Isn T The Answer 2 Quora




Evaluate Root 3 2root2 Evaluate Root 3 Plus 2 Root 2 Sqrt 3 2 Sqrt2 Youtube




Find The Value Of A And B If 3 2 3 2 A B 2 Brainly In
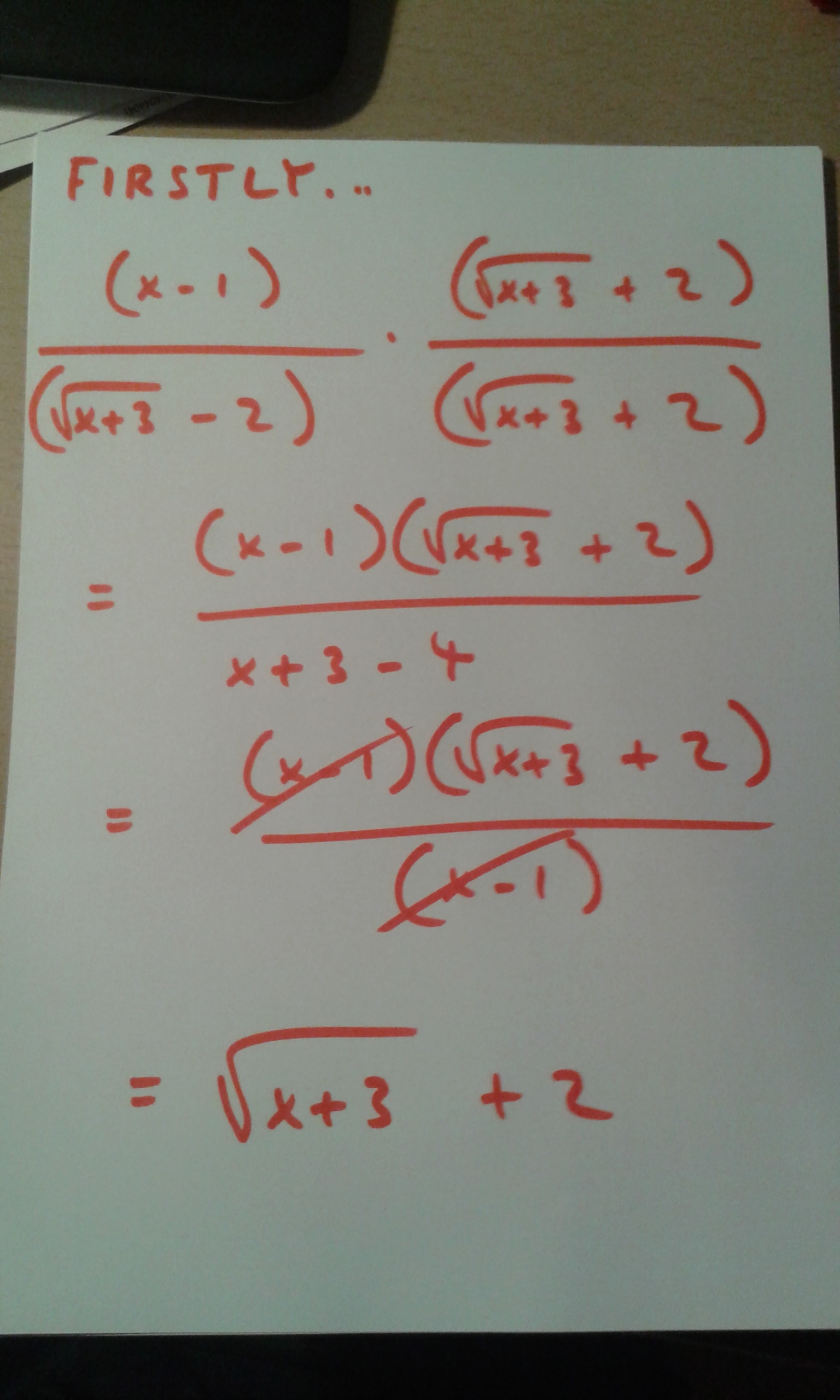



How Do You Find The Limit Of X 1 Sqrt X 3 2 As X Approaches 1 Socratic




3 2 4 2 12 32 Simplify The Product Brainly In




Find The Value Of2 3 8 18 Brainly In




After Rationalizing The Denominator Of 2 3 5 2 2 3 3 And Simplifying We Get A 3 15 B 10 4 6 19 Then The Value Of A B Is




3 2 Square Root 3 By 3 Minus 2 Square Root 3 Is Equal To A B Square Root 3 Find Value Of A B All 3 Brainly In
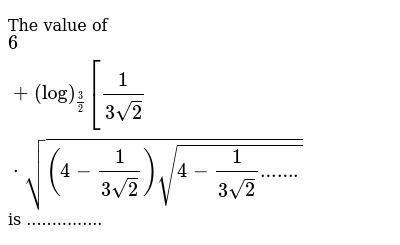



The Value Of 6 Log 3 2 1 3sqrt 2 Sqrt 4 1 3sqrt 2



If X 3 2 2 Then What Is The Value Of X 1 X Quora




If X 3 2 3 2 Y 3 2 3 2 Find The Value Of X 2 Y 2 Xy Mathematics Topperlearning Com 9dqpqijj




If X 2 3 Find The Value Of X 2 1 X 2 If X 2 3 Find The Value Of X2 1 X2 Polynomials Class 9 Youtube



How To Solve This 3 1 3 1 Quora




If X 3 2 2 Then Check Whether X 1 X Is Rational Or Irrational Brainly In



What Is The Sin 1 Sqrt 3 2 Socratic




If A 3 2 Root 2 Then Find The Value Of A 2 1 A 2 Brainly In
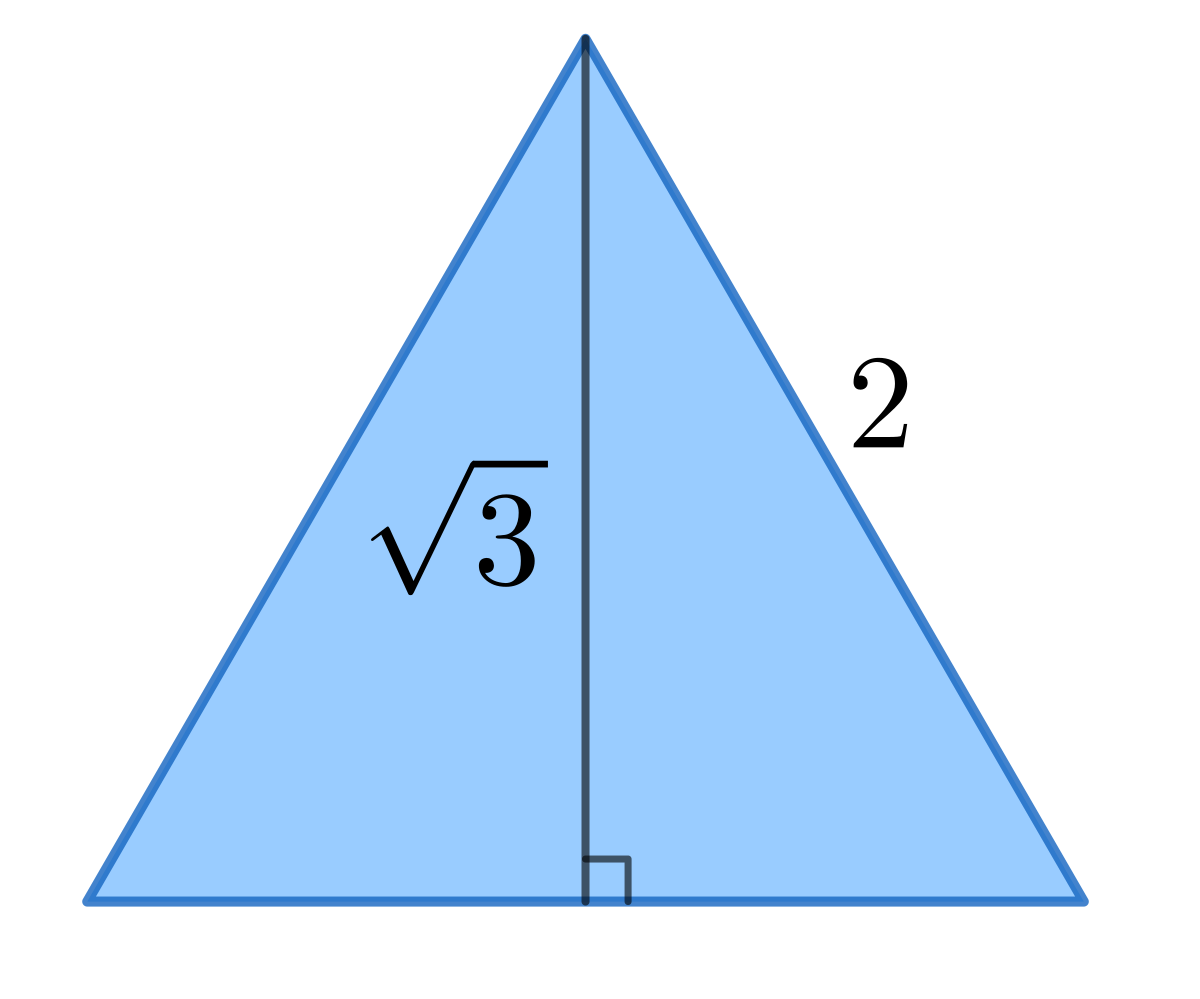



Square Root Of 3 Wikipedia
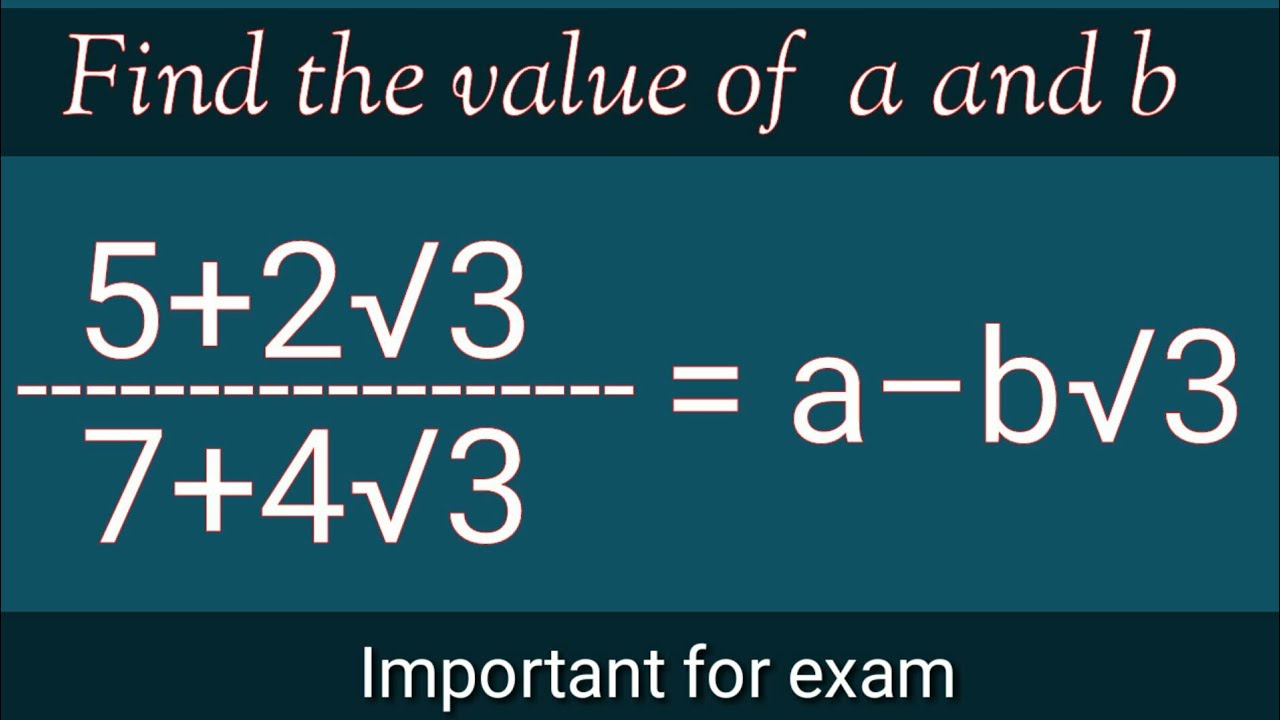



Find The Value Of A And B 5 2 3 7 4 3 A B 3 Youtube
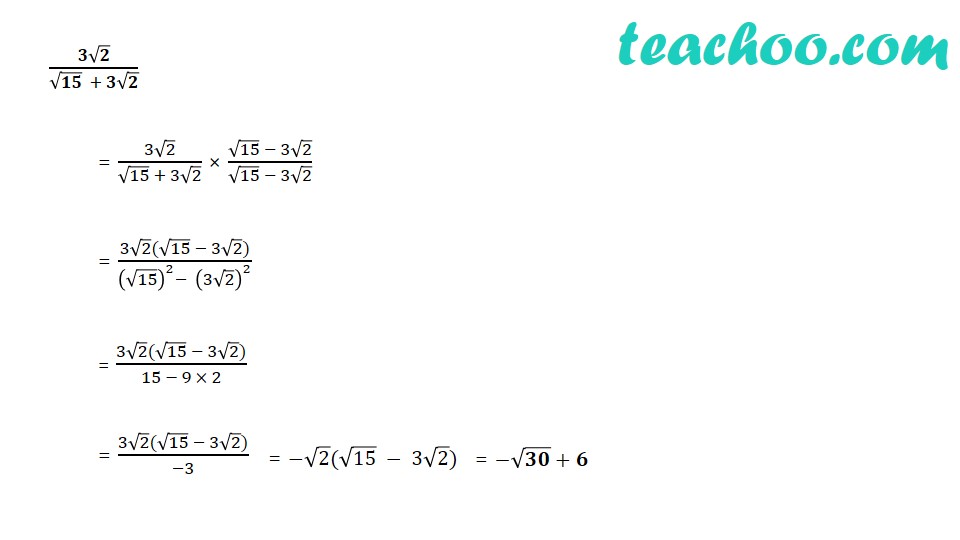



Simplify 7 3 10 3 2 5 6 5 3 2 15 3 2 V



What Is The Value Of 3 2 2 Quora




If X 3 2 2 Find The Value Of X3 1 X3 Brainly In



0 件のコメント:
コメントを投稿